Solving System Of Linear Differential Equations
Solving system of linear differential equations. Recall that a linear equation can take the form latexAxByC0latex. For example is a system of three equations in the three variables x y zA solution to a linear system is an assignment of values to the variables such that all the equations are simultaneously satisfied. B 1 x b 2 y b 3 z d 2.
Those systems of two equations and two unknowns only. Modeled on the MIT mathlet Amplitude and Phase. Lets see some examples of first order first degree DEs.
Linear differential equations with variable coefficients and Mittag-Leffler kernels. All New SAT Math - Calculator Resources. Use known DE types to model and understand situations involving exponential growth or decay and second order physical systems such as driven spring.
The aim of this tutorial is to give an introductory overview of the finite element method FEM as it is implemented in NDSolve. It can handle a wide range of ordinary differential equations ODEs as well as some partial differential equations PDEs. Systems of linear equations arise naturally in many real-life applications in a wide range of areas such as in the solution of Partial Differential Equations the calibration of financial models fluid simulation or numerical field calculation.
Find the general solution for the differential equation dy 7x dx 0 b. Introduction to Advanced Numerical Differential Equation Solving in Mathematica Overview The Mathematica function NDSolve is a general numerical differential equation solver. Data-driven solution and data-driven discovery of partial differential.
This is also true for a. Perhaps the best method to solve systems of linear inequalities is by graphing the inequalities. A linear differential equation or a system of linear equations such that the associated homogeneous equations have constant coefficients may be solved by quadrature which means that the solutions may be expressed in terms of integrals.
We substitute A L U. A 1 x a 2 y a 3 z d 1.
Introduction to Advanced Numerical Differential Equation Solving in Mathematica Overview The Mathematica function NDSolve is a general numerical differential equation solver.
These equations can be written as. B 1 x b 2 y b 3 z d 2. A 1n x n g 1 x 2 a 21. Systems of linear equations arise naturally in many real-life applications in a wide range of areas such as in the solution of Partial Differential Equations the calibration of financial models fluid simulation or numerical field calculation. We introduce physics-informed neural networks neural networks that are trained to solve supervised learning tasks while respecting any given laws of physics described by general nonlinear partial differential equations. For these equations students will be able to. This is also true for a. Separable Equations Identifying and solving separable first order differential equations. But first we shall have a brief overview and learn some notations and terminology.
In a system of ordinary differential equations there can be any number of. Matrix Method of Solving Linear Equations. Results on system of Atangana-Baleanu fractional order Willis aneurysm. We substitute A L U. A 1 x a 2 y a 3 z d 1. Linear differential equations with variable coefficients and Mittag-Leffler kernels. Periodic response of a second order system.









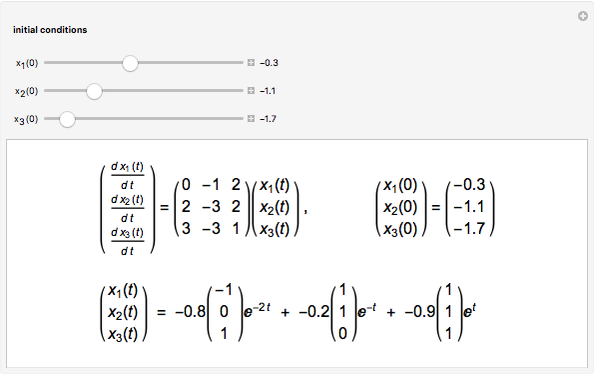




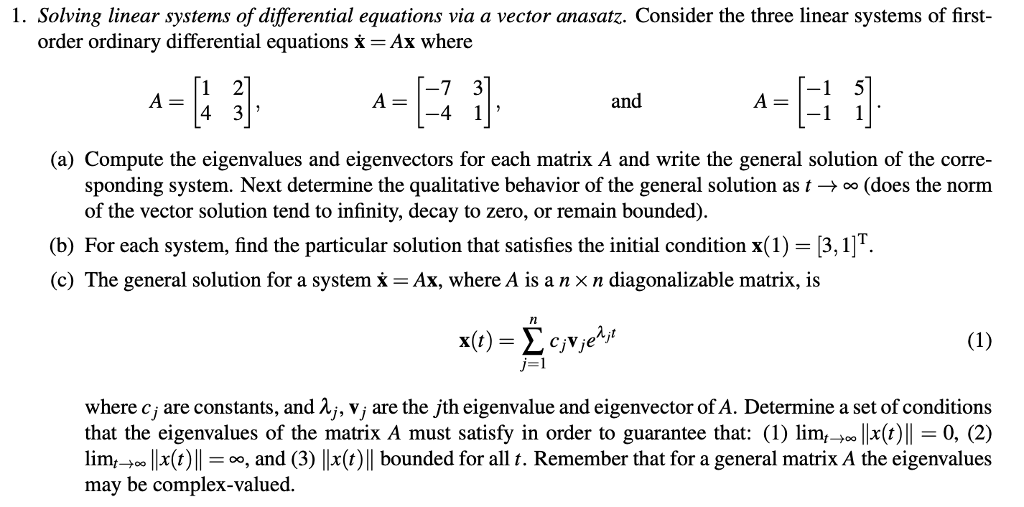
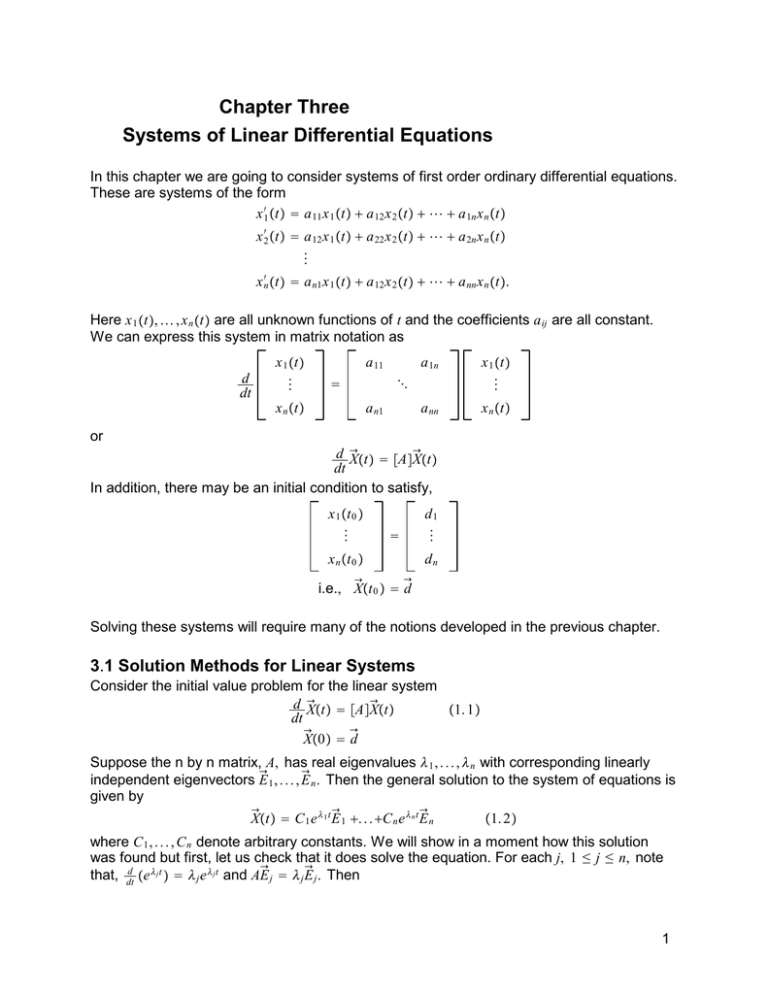


















Post a Comment for "Solving System Of Linear Differential Equations"